${\mathit {\mathit d}}$-QUARK MASS
INSPIRE JSON (beta) PDGID:Q123DM
See the comment for the ${{\mathit u}}$ quark above.
We have normalized the $\overline{\rm{}MS}$ masses at a renormalization scale of $\mu $ = 2 GeV. Results quoted in the literature at $\mu $ = 1 GeV have been rescaled by dividing by $1.35$. The values of “Our Evaluation” were determined in part via Figures$~$2 and 3 in the “Quark masses” review.
We have normalized the $\overline{\rm{}MS}$ masses at a renormalization scale of $\mu $ = 2 GeV. Results quoted in the literature at $\mu $ = 1 GeV have been rescaled by dividing by $1.35$. The values of “Our Evaluation” were determined in part via Figures$~$2 and 3 in the “Quark masses” review.
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
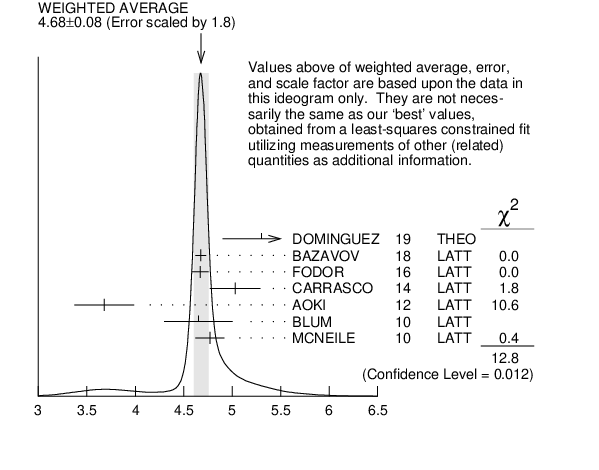
${\mathit {\mathit d}}$-QUARK MASS (MeV) | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| References | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Except where otherwise noted, content of the 2025 Review of Particle Physics is licensed under a Creative Commons Attribution 4.0 International (CC BY 4.0) license. The publication of the Review of Particle Physics is supported by US DOE, MEXT (Japan), INFN (Italy) and CERN. Individual collaborators receive support for their PDG activities from their respective institutes or funding agencies. © 2025. See LBNL disclaimers.